[abonnement gratuit]

the author

  Download PDF

  en français
 

Contents

• I  Plane Mirrors

• II  Spherical Mirrors

• III  Prism

• IV  Plane-Parallel Plate

• V  Spherical Refracting Surface

• VI  Thin Lens Elements

  • VI 1  Positive Lens Elements

  • VI 2  General Algebraic Formulae

  • VI 3  Combination of Two Thin Lens Elements

• VII   Measurement of Focal Lengths

  • VII 1  Cornu’s Method

  • VII 2  Simplified Method to Find the Focal Length of a Thick Positive Lens

• VIII   Classical Visual Instruments

  • VIII 1   The Human Eye

  • VIII 2   Loupe, Magnifying Glass

  • VIII 3   Microscope

  • VIII 4   Astronomical refractor telescope

  • VIII 5   Binoculars

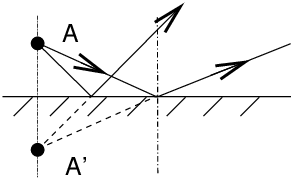
  • VIII 6   Appendix: Adjustment of instruments to avoid visual strain

Introduction

The practice of fully automatic cameras, of which the mobile phone may be an emblematic example in amateur photography at the beginning of the 21st century, naturally takes the photographer away from the old principles of optical image formation, and when moving to medium format or large format film cameras, and even more when starting a do-it-yourself camera construction project – e.g. a large format self-built camera, an exciting challenge – this will raise many questions to which discussion forums try to answer.

Of course, it is the rule to practice medium or large format without ever using the equations and diagrams of academic courses! The purely manual, visual and tactile link of the photographer with a camera devoid of any automatism seems at a first glance incompatible with a kind of intellectual approach that would like to model everything and calculate all settings in advance... But on the other hand, the number of frequently asked technical questions on image formation, lenses, sharpness, depth of field and diffraction effects on discussion forums shows us that a quick look at good books and basic training courses in optics will never harm an artistic practice.

The purpose of this formulary is therefore to encourage readers who want to go further in their practice of medium and large format cameras – practitioners of smaller film formats and digital cameras being also welcome!!!– to reread classic textbooks in geometrical or instrumental optics, and to keep this formulary handy to quickly find a formula or a diagram.

I  Plane Mirrors

The image A’ is symmetrical from A with respect to the mirror plane.

---

Figure 1: The image in a plane mirror is symmetrical of the object with respect to the plane of the mirror.

---

Successive images given by a combination of plane mirrors can be constructed geometrically

• even number of mirrors = rotation + translation

• odd number of mirrors = symmetry + translation

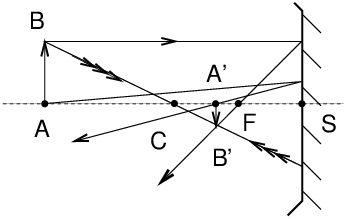
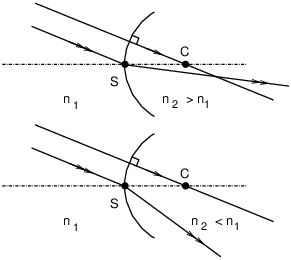
II  Spherical Mirrors

Paraxial algebraic formulae: e.g. CS=−SC

---

Converging spherical mirror, paraxial constructions

---

An incident ray parallel to the axis passes through the focal point F after reflection; a ray passing through the centre C returns back to itself after reflection.

CS=R SF=f=SC/2

formulae with origins at the mirror centre C    1/CA+1/CA′=2/CS

formulae with origins at the mirror vertex S    1/SA+1/SA′=2/SC

image magnification M = −f/FA=−FA′/f FA × FA′=f²

III  Prism

---

Figure 2: Deflection of light by a prism, general case

---

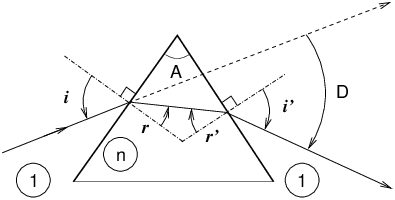
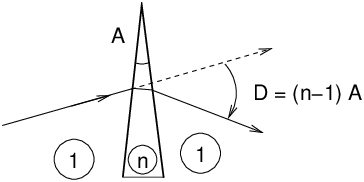
Angle of the prism A, refractive index n, deflection D, fundamental equations

A minimum of deflection exists in the symmetrical configuration where i=i′ and r=r′.
In this particular case we get

the index of the prism is deduced from it, which allows measurements of n.

Prism with a small angle

---

Figure 3: Light deflection by a prism with a small angle

---

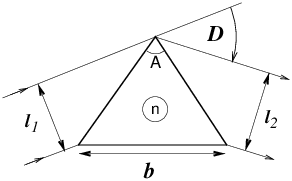
In this case, we have the approximate relation: D=(n−1)A. Even if the prism is of low angle, and even more in the general case, a prism is only stigmatic for parallel rays at the entrance, in other words a source point at infinity. A source point at a finite distance will give a blurred image at the output. Moreover, in polychromatic lighting, the dispersion of colours further worsens the phenomenon.

Dispersion: the refractive index n depends the wavelength of light λ, and therefore D depends on λ.

---

Figure 4: Relation between the dispersion of the prism and its geometry

---

Taking into account that dn/dλ depends on glass properties, it follows

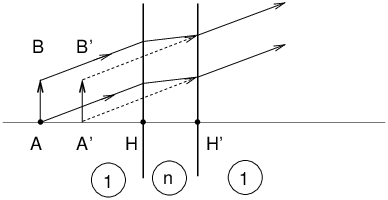
dD/dn can be calculated geometrically and is equal to b/l₂.

IV  Plane-Parallel Plate

---

Figure 5: The effect of a plane-parallel plate is a constant translation between the object and the image

---

Example: air/glass : n=3/2 AA′ ≃ 1/3 HH′

V  Spherical Refracting Surface

Lagrange-Helmholtz Formula

---

Figure 6: Paraxial object-image ray tracing in a spherical refracting surface

---

In a spherical refracting surface

• The refracting surface only exists if refractive indices n₁ and n₂ are different!

• Focal lengths f₁ and f₂ are therefore different;

• Any light ray passing through the centre C is not deflected;

• But any ray passing through the vertex S is deflected! (Be careful, do not make a confusion with what happens in a thin lens element)

---

Figure 7: Ray tracing for a spherical refractive surface, according to the refractive indices of the media

---

Formulae with origins at the vertex S

Formulae with origins at the centre C (please note how indices 1 and 2 are swapped with respect to the other formula with origins a the vertex)

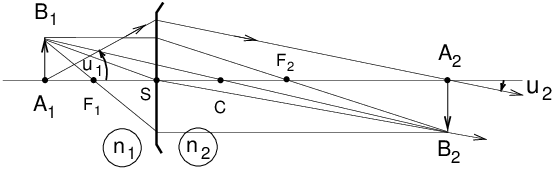
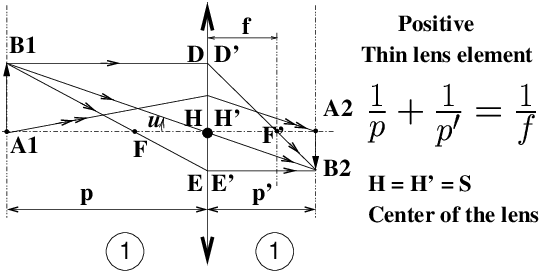
VI  Thin Lens Elements

VI 1  Positive Lens Elements

Descartes’ formulae “arithmetic” (all positive quantities) for photographic lenses, or other photographic applications, projection of slides or transparencies with a real object A₁ and a real image A₂ read as follows

---

Figure 8: Object-image ray tracing for a thin positive lens element

---

In order for the object-image conjugation to take place, it can be shown that a minimum object-image distance is required

Examples: to make a projection with a positive lens of 250 mm focal length it is impossible to focus if it there is less than 1m between the object and the screen. For a 35-mm slide projector equipped with a lens of 90 mm focal length, this minimum distance is reduced to 360 mm. At the minimum distance A₁ A₂ = 4 f we are at a 1:1 ratio, this situation has little interest for a projection (except for image transport with image inversion in an optical instrument like old-style spyglasses), but which corresponds to the macro-photo situation with the image of the same size as the object. Shooting in this configuration called "2f-2f" corresponds to the 1:1 ratio (image size = object size) which allows to photograph a full-frame postage stamp in 35-mm format. Starting from a camera set-up for conventional landscape photography at large distances, object to infinity, image in the focal plane, in order to reach the 1:1 ratio, it will be necessary to extend the lens-to-film distance by an amount equal to one focal length, using one or more extension rings or a macro bellows (see below "Measurement of focal lengths").

VI 2  General Algebraic Formulae

For any centred system, within the Gauss approximation.

---

Figure 9: Focal points and principal planes of a thick centred system

---

Generalised Descartes formulae with origins at the principal planes H and H′; if it is necessary to remember only one algebraic formula, valid in all cases (real or virtual objects, positive or negative lenses, association of thin or thick lens elements), prefer this one

where C denotes the convergence of the system, with

Principal points H and H′ are points on the optical axis located in the principal planes. Principal planes are object/image conjugated planes with a magnification equal to +1. Principal planes are found by looking for the intersection of an incident light ray parallel to the optical axis with the corresponding emerging ray (this determines the image principal plane passing through H′), or by finding the intersection of an emerging parallel ray with the corresponding incident ray (this determines the object principal plane passing through H).

Object / image lateral magnification

Generalised Newton’s formulae

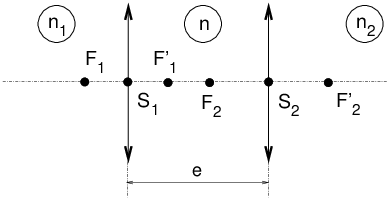
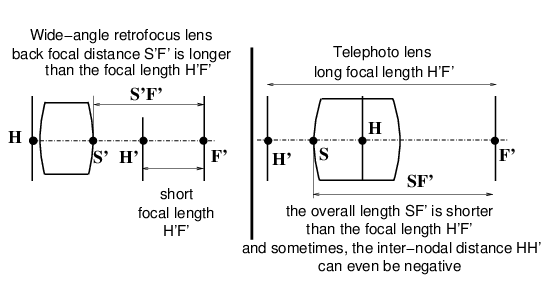
Nodal points N and N′: nodal points of a thick compound optical system are two points on the optical axis, conjugate of each other, and for which corresponding rays cross the axis at those points with the same angle w/respect to the axis (the angular ratio is equal to +1). In optical systems where refractive indices are the same on input and output, e.g. for any usual photographic application in air, nodal points are identical to principal points, i.e. N=H and N′=H′.

In such a system, the most common ones in photography, the object focal length and the image focal length are equal in magnitude and of opposite sign i.e.: HF = −H′F′. Any system with identical refractive indices on input and output has only one focal length, f′=H′F′ which is positive for a positive system (most photographic lenses) and negative for a negative system (e.g. a teleconverter, a doubler).

VI 3  Combination of Two Thin Lens Elements

Consider the combination of two thin lens elements, with input and output indices n₁ and n₂, with an intermediate index n between the two elements.

---

Figure 10: Combination of two thin lens elements to build a thick compound lens

---

Define as C₁ and C₂ the convergences, being equal to the inverse of focal lengths (object or image focal length), multiplied by the corresponding refractive index (input or output) of each thin lens element.

The convergence C of the combined system is given by Gullstrand’s formula

Note: the location of principal planes H and H′ can be anywhere and without any obvious relationship between the location of the centres of thin lens elements.

Simplest case: two thin lens elements stacked one over each other without gap (e=0) ⇒ C=C₁+C₂. In air (n₁=n₂=1) this yields 1/f = 1/f₁ + 1/f₂

VII   Measurement of Focal Lengths

VII 1  Cornu’s Method

---

Figure 11: Determination of principal planes and focal lengths of a thick compound lens using Cornu’s method

---

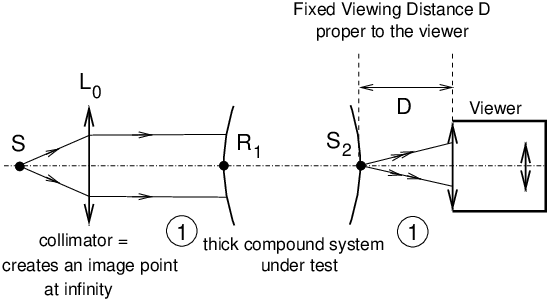
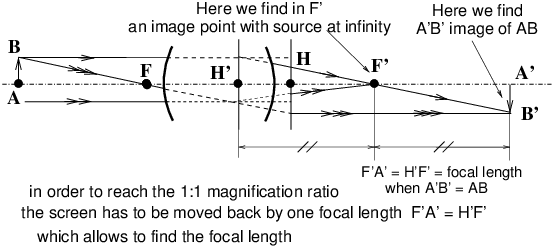
A light source S is located at the object focal point F of a positive lens L₀. This set-up is named: collimator.

Measurements require an optical bench and a fixed-distance viewer able to precisely focus through an eyepiece at various image locations as explained below. We assume that the optical axis is horizontal.

• Collimator set-up: for example by auto-collimation with a plane mirror located close to the exit side of L₀, reflecting light back to S. The proper set-up is obtained when the reflected image exactly coincides with S.

• When the collimator is properly set-up, the image of S through L₀ is at infinity, hence S→ ∞ → yields through the lens under test an image F′=F₂, i.e. the image focal point of the system. Now various images are to be located using the fixed-distance viewer.

  • focus to S₂, the exit lens vertex of the system (e.g. place a small piece of paper on the lens vertex and focus on it)

  • focus to R₂ image of R₁ through the system

• rotate the system by 180° around a vertical axis so that input becomes exit and vice-versa: S now yields the object focal point of the system F=F₁

  • focus to R₁

  • focus to S₁ the image of S₂ through the system

• compute the following (positive or negative) distances F₂ S₂, F₂ R₂, F₁ R₁, F₁ S₁

• Newton’s formulae yield: HF₁× H′F₂= −f′²= F₁ R₁× F₂ R₂= F₁ S₁× F₂ S₂

Now we have the location of foci F=F₁, F′=F₂ and the focal lengths HF₁ = −H′F₂ = −f′, hence we locate H and H′, at a distance of one focal length f′ from the corresponding foci.

VII 2  Simplified Method to Find the Focal Length of a Thick Positive Lens

---

Figure 12: Simplified method to find the focal length of an unknown thick positive lens, infinity -> focus and 1:1 magnification ratio

---

This method is valid for all positive lenses, e.g . photographic lenses. In old French textbooks, it is know as Davanne & Martin’s method (end of the XIX-st century).

Note: the diagram represents H behind H′, this situation may exist for a thick compound lens.

• collimator + parallel beam ⇒ F′ focused on screen

• the distance between the system and the screen is set-up so that the object AB → yields an image at A′B′ of same size as AB but reversed on the screen. ¹

• ⇒ in this situation, object and image are conjugated in the “2f-2f” setting ⇒ F′ A′ = H′ F′=f′.

• The required distance, moving the screen backward beyond the image focal point F′, allowing to focus at 1:1 ratio (“2f-2f”) is equal to f′ for any thick positive compound lens of any design, the location of principal points H and H′ can be anywhere.

This method is relevant to any photographic lens, providing it is a positive system (and not a tele-converter which is a negative system).

---

Figure 13: Principal planes in photographic wide-angle retrofocus lenses and telephoto lenses

---

VIII   Classical Visual Instruments

VIII 1   The Human Eye

In principle a “normal” human eye sees sharp at infinity without accommodating.

• A short-sighted eye can see sharp only at short distances. For example this distance can be D = 10 cm (quite severely short-sighted, but can be corrected with a negative lens of 10 dioptres) or 1 m (correction of 1 dioptre, weakly short-sighted).

  A hyperopic (long-sighted) eye must make an effort to accommodate even to see sharp at infinity. This yields an uncomfortable eye strain (accommodation) to write or read at distance D = 30 cm.

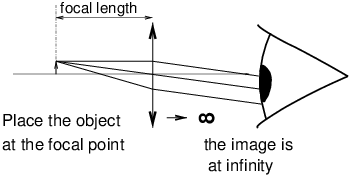
VIII 2   Loupe, Magnifying Glass

The object is located at the object focus of the magnifying glass, ⇒ the image is sent to infinity.

---

Figure 14: Classical focus->infinity set-up for a magnifying glass

---

The intrinsic “commercial” magnification of a loupe is frequently défined as: M_ic=1/4f′, where f′ is the focal length in meters.
For example, the commercial engraving “8×” means: M_ic=8 f′~ 3,1 cm.

VIII 3   Microscope

---

Figure 15: Principles of the Optical Microscope

---

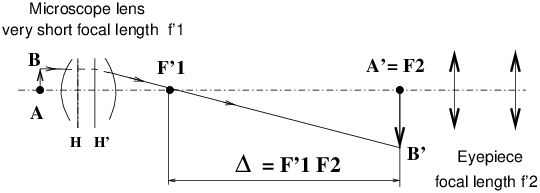
A′ B′ is the magnified image of A B. A microscope lens is a positive optical system with a large convergence (short focal length f′₁, from a few mm to one cm). The eyepiece is a separate optical system of positive focal length sending the magnified image A′B′ delivered by the microscope lens to infinity for visual observation. 

In those conditions, the observer can see sharp without accommodating. The distance (“tube length”) F′₁ F₂ ~ 16 cm in classical microscopes (for biological samples). The microscope power P is defined as: P≃ Δ/f′₁ f′₂. The magnification M_obj. (for the microsope lens) is given by M_obj.=Δ/f′₁. The intrisinc commercial magnification of the microscope M_cm is given by M_cm=M_obj.× M_eyep.. The orders of magnitude are: M_cm from 25 to 2500, M_obj. from 2.5 to 120, and for eyepieces M_eyep. from 5 to 20.

VIII 4   Astronomical refractor telescope

---

Figure 16: Principles of the refractor astronomical telescope in the “afocal” set-up, location of the exit pupil, where the eye should be

---

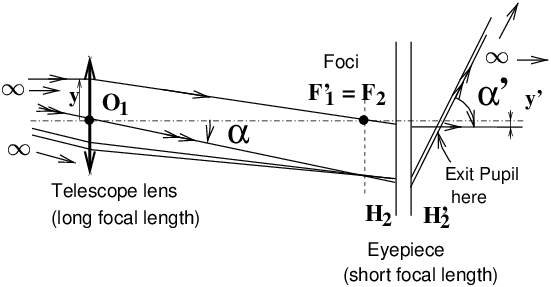
The telescope is set-up in the afocal configuration or ∞−∞ set-up, if F′₁ and F₂ coincidate. The angular magnification M_α is given by

In such an afocal system, the lateral magnification M_y is independent from the object’s position. M_y is given by

Typical values for M_α : f′₁=1 m, f′₂=2 cm ⇒ M_α=−50 (minus sign, the image is reversed).

VIII 5   Binoculars

A pair of binoculars is equivalent to two coupled astronomical glasses, combined with erecting prisms to restore the image "in the right direction". Meaning of commercial indications: 8 × 20 means 8 for the angular magnification (without unit) and 20 for the diameter of the lens expressed in mm. Above G ≃ 10 you don’t gain anything in terms of image details for freehand use anymore, because of the motion blur².

By dividing the diameter of the lens (e.g. 20 mm) by the magnification (e.g. 8) the diameter of the "exit pupil", i. e. the diameter of the light beam just outside the eyepiece, i.e. 2.5 mm in the selected example, is obtained. For use of the binoculars by day light, an exit pupil of 2.5 mm is sufficient. With binoculars 8 × 50, the exit pupil will be 6.25 mm which is only useful for use at night. Indeed during the day the pupil of the human eye does not exceed a diameter of about 2.5 mm; therefore this pupil size actually limits the diameter of the beam: it is useless to pay a very expensive price for a 8 × 50 instrument in this case. At night, on the other hand, since the pupil of the human eye is at least opened by 6 mm in diameter, we will benefit from a "light funnel" effect, for night scenes or star observation³.

VIII 6   Appendix: Adjustment of instruments to avoid visual strain

PURPOSE: to ensure that the observed image is "far ahead" and not between 1 and 20 cm... which would lead to forced accommodation, especially embarrassing when both eyes do not observe at the same distance, when the instrument is out of proper focusing.

For all binocular instruments, it is essential to set the inter-pupil distance correctly, as no operator is built in the same way! Modern wide-field eyepieces make it possible to keep corrective eyeglasses on; this is important, especially for the correction of astigmatism, which can only be achieved by ophthalmic lenses. Weak myopia or hyperopia without astigmatism, on the other hand, can be easily compensated by adjusting the central sharpness setting and diopter correction of either eyepiece (see below).

• Binocular magnifier, low power microscope:

  Move the lens + eyepiece assembly away from the object, then gradually move closer without exceeding optimal focus. If we go beyond this point, in very little movement of the microscope the image placed in principle at infinity approaches below ≃ 20cm, which leads to forced accommodation.

  Disadvantage with high-power microscopes, which have a very short frontal viewing distance (1 mm or less): with this method there is a risk of damaging the object or breaking the slide cover (thickness: 0.16 to 0.18 mm) by approaching too close. In elementary classes, natural science teachers recommend that the microscope be placed in contact with the object or the cover blade first, then gradually moved away. This second method reduces the risk of breakage of the equipment. If this is done, it is then preferable for the eyes to exceed the sharpness point and "re-lower" to resume focusing without exceeding the optimum. In all these fine adjustments it is preferable to keep grabbing the focusing screw with fingers so that you can easily return to a sharp position that often passes very quickly.

• Binoculars, astronomical telescope:

  Keep the eyepieces as far away from front lenses as possible by playing with the central adjustment screw. Move closer gradually without exceeding optimal focus. In general, one of the eyepieces has an additional adjustment to compensate for any diopter gap between the two eyes; in this case, make the adjustment as previously on the side of the fixed eyepiece with the central screw, then adjust the adjustable eyepiece "in the right direction", i. e. by moving away from front lenses and then moving the eyepiece closer (unscrew, then screw in...).

• Modern binoculars with internal focusing:

  Modern quality binoculars often have an "internal" focusing mechanism. It is impossible to see if the eyepieces are moving away from or closer to front lenses, since the eyepieces are focused by moving a group of lenses internally.
  In this case, it is preferable to proceed as follows:

  • Roughly adjust the sharpness on a "near" object (5 to 10 m)

  • Point binoculars to the distant object you are looking for; to recover the sharpness from the previous focus, we are then forced to move the setting "in the right direction", the best for the accommodation of the eye. Again, do not exceed the point of better sharpness.

See other articles on www.galerie-photo.com

Questions, comments?

• Please send an e-mail to the author:
  http://bigler.blog.free.fr/public/images/signature-eb-forums.jpg
• Ask a question on the galerie-photo.info forum:
  http://www.galerie-photo.info/forumgp

1	Note: this is only possible if the object-image distance is greater than 4f′, hence if the focal length of the system f′ is totally unknown, one should allow as much space as possible between the object and the screen.
2	with the notable exception of some recent models equipped with an opto-mechanical anti-shake system inspired by what is used in some telephoto lenses.
3	for a precise discussion of the possible gains in "luminosity" when comparing observation with the naked eye and observation through an instrument, see the classic textbooks. The result is not at all intuitive.

version 4th November, 2019